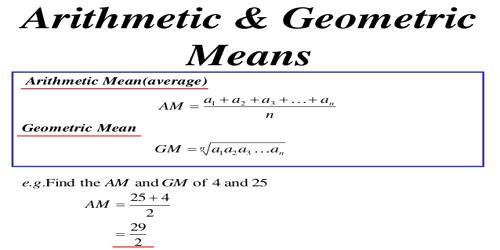
The arithmetic mean, also called the average or average value, is the quantity obtained by summing two or more numbers or variables and then dividing by the number of numbers or variables. The arithmetic mean is important in statistics.
The arithmetic mean of a continuous function over a defined interval is determined by first calculating the definite integral over the interval, and then dividing this quantity by the width of the interval.
The geometric mean is a type of average, usually used for growth rates, like population growth or interest rates. While the arithmetic mean adds items, the geometric mean multiplies items. Also, you can only get the geometric mean for positive numbers. If three quantities are in Geometric Progression then the middle one is called the geometric mean of the other two.
Relation between Arithmetic Means and Geometric Means
The following properties are:
Property- I: The Arithmetic Means of two positive numbers can never be less than their Geometric Mean.
Proof:
Let A and G be the Arithmetic Means and Geometric Means respectively of two positive numbers m and n.
Then, we have A = m + n/2 and G = ±√mn
Since m and n are positive numbers, hence it is evident that A > G when G = -√mn. Therefore, we are to show A ≥ G when G = √mn.
We have, A – G = m + n/2 – √mn = m + n – 2√mn/2
A – G = ½[(√m – √n)^2] ≥ 0
Therefore, A – G ≥ 0 or, A ≥ G.
Hence, the Arithmetic Mean of two positive numbers can never be less than their Geometric Means. (Proved).
Property- II: If A be the Arithmetic Means and G be the Geometric Means between two positive numbers m and n, then the quadratic equation whose roots are m, n is x^2 – 2Ax + G^2 = 0.
Proof:
Since A and G be the Arithmetic Means and Geometric Means respectively of two positive numbers m and n then, we have
A = m + n/2 and G = √mn.
The equation having m, n as its roots are
x^2 – x(m + n) + nm = 0
⇒ x^2 – 2Ax + G^2 = 0, [Since, A = m + n/2 and G = √nm]
Property- III: If A be the Arithmetic Means and G be the Geometric Means between two positive numbers, then the numbers are A ± √A^2 – G^2.
Proof:
Since, A and G be the Arithmetic Means and Geometric Means respectively then, the equation having its roots as the given numbers is
x^2 – 2Ax + G^2 = 0
⇒ x = 2A ± √4A^2 – 4G^2/2
⇒ x = A ± √A^2 – G^2
Property IV: If the Arithmetic Mean of two numbers x and y is to their Geometric Mean as p : q, then, x : y = (p + √(p^2 – q^2) : (p – √(p^2 – q^2).
Solved examples of the properties of Arithmetic and Geometric Means between two given quantities:
- The Arithmetic and Geometric Means of two positive numbers are 15 and 9 respectively. Find the numbers.
Solution:
Let the two positive numbers be x and y. Then according to the problem,
x + y/2 = 15
or, x + y = 30 ……………… (i)
and √xy = 9
or xy = 81
Now, (x – y)^2 = (x + y)^2 – 4xy = (30)^2 – 4 * 81 = 576 = (24)^2
Therefore, x – y = ± 24 ……………… (ii)
Solving (ii) and (iii), we get,
2x = 54 or 2x = 6
x = 27 or x = 3
When x = 27 then y = 30 – x = 30 – 27 = 3
and when x = 27 then y = 30 – x = 30 – 3 = 27
Therefore, the required numbers are 27 and 3.
- Find two positive numbers whose Arithmetic Means increased by 2 than Geometric Means and their difference is 12.
Solution:
Let the two numbers be m and n. Then,
m – n = 12 …………………… (i)
It is given that AM – GM = 2
⇒ m + n/2 – √mn = 2
⇒ m + n – √mn = 4
⇒ (√m – √n^2 = 4
⇒ √m – √n = ±2 …………………… (ii)
Now, m – n = 12
⇒ (√m + √n)(√m – √n) = 12
⇒ (√m + √n)(±2) = 12 …………………… (iii)
⇒ √m + √n = ± 6, [using (ii)]
Solving (ii) and (iii), we get m = 16, n = 4
Hence, the required numbers are 16 and 4.
- If 34 and 16 are the Arithmetic Means and Geometric Means of two positive numbers respectively. Find the numbers.
Solution:
Let the two numbers be m and n. Then
Arithmetic Mean = 34
⇒ m + n/2 = 34
⇒ m + n = 68
And
Geometric Mean = 16
√mn = 16
⇒ mn = 256 …………………………. (i)
Therefore, (m – n)^2 = (m + n)^2 – 4mn
⇒ (m – n)^2 = (68)^2 – 4 × 256 = 3600
⇒ m – n = 60…………………………. (ii)
On solving (i) and (ii), we get m = 64 and n = 4.
Hence, the required numbers are 64 and 4.
Information Source;