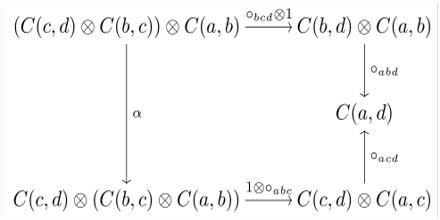Enriched Category Theory thus encompasses within the same framework a wide variety of structures including ordinary categories where the hom-set carries additional structure beyond being a set. It’s like entities that don’t themselves have any notion of individual morphism but whose hom-objects have similar compositional aspects. It is the idea of a category by replacing hom-sets with objects from a general monoidal category. In an enriched category, the set of morphisms associated with every pair of objects is replaced by an opaque object in some fixed monoidal category of “hom-objects”.
Enriched Category Theory