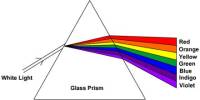
Principle purpose of this lecture is to discuss on Time Travel in Physics and Science Fiction. We all can travel through time but always forward and we also can communicate through time but always from past to future. Relativity tells us how to move forward through occasion more slowly go fast or search for a high-gravity region, and even how to stop time altogether search for a black hole’s event horizon. Finally discuss on the Law of Causality, Time Travel Paradoxes in SF and Time Travel in Physics.
Time Travel in Physics and Science Fiction